Margin of error is a statistic that states the number of errors in random sampling in a survey. Margin of error can also be defined as a distance in a certain confidence interval (statistical) from the survey, this distance represents a statistic. Margin of error measures how close the results of the sample are to the results in reality.
In statistic, we calculate the confidence interval to see where the value of the data of sample statistic will fall. The range of values which are below and above the sample statistic in a confidence interval is known as Margin of Error. In other words, it is basically the degree of error in the sample statistic.
Higher the margin of error, lesser will the confidence in the results because the degree of deviation in these results is very high. As its name suggests, the margin of error is a range of values above and below the actual results. For example, if we get a response in a survey wherein 70% people have responded “good” and margin of error is 5%, this means that in general, 65% to 75% of the population think that the answer is “good”.
Calculating Margin of Error
In calculating the error margin, the factors that influence are:
- Sample size
- The importance of problems in the sample
- Statistics or estimators themselves
The larger the sample will increasingly represent the population. But we know in taking samples and the sample itself is always biased. For that we use margin error to reduce the bias that occurs. Bias that can be known from the margin error is the bias arising from the sampling error.
Standard error of proportion and confidence interval.
To find out the margin error calculation, it must first be known about the standard error of proportion and confidence interval.
Confidence interval (CI) is a percentage that describes how close the original value is to the statistics (estimator) measured +/- margin error.
Standard error of proportions measures the accuracy of the estimator of proportions and estimates the standard deviation of percentages.
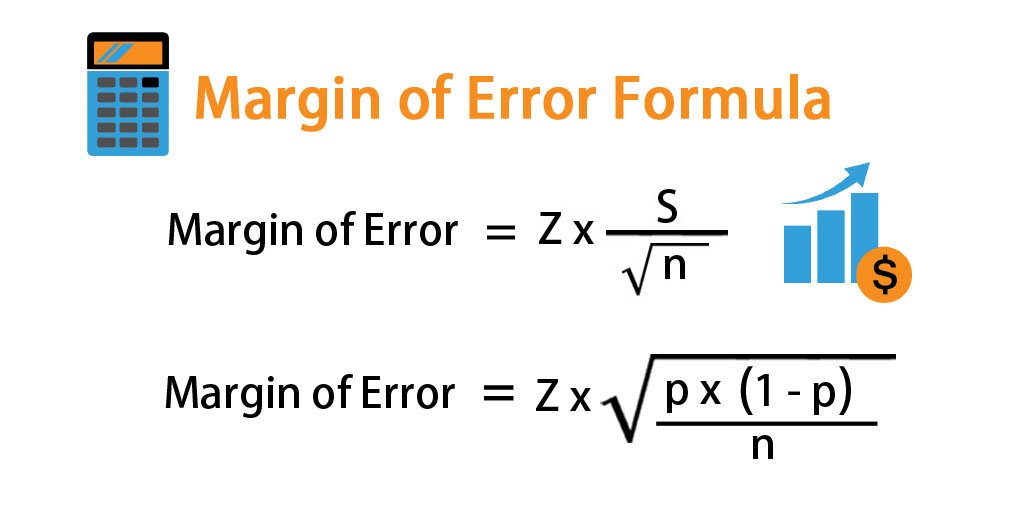
It can be predicted from p and the size of sample n, if n is small enough then use the formula:
Standard error = √p(1-p)/n
To estimate the proportions of plus and minus, the margin error is the confidence interval for the proportion or percentage. In other words, the error margin is part of the confidence interval. The error margin is calculated by multiplying the standard error with the confidence factor of a certain level of confidence. Example:
- The margin (plus – minus estimator) of 1 standard error is 68% confidence interval,
- The margin (plus – minus estimator) of 1.96 standard error is 95% confidence interval
- The margin (plus – minus estimator) of 2.58 standard error is 99% confidence interval
Maximum margin error
The maximum margin of error for proportions is the distance from the confidence interval when p = 50%. For 95% confidence, the formula is
(maximum) error margin (95%) = 1.96 x √0.5 (1-0.5) / n = 0.98 / √n
Different confidence intervals
The margin error depends on the probability of a confidence interval, like this:
- Error margin (99%) confidence = 1.29 / √n
- Error margin (95%) confidence = 0.98 / √n
- Error margin (90%) confidence = 0.82 / √n
Some mistakes people make in understanding the use of margin errors:
- Do not use or do not involve confidence intervals. You will not know what your error margin is if the confidence interval is unknown
- Many people believe that the error margin represents the entirety of the error. Even though this is not true, what is included in the margin error is the sampling error and the non sampling error cannot be seen from the margin error.
- People sometimes believe that the margin error indicates the quality of the survey, meaning that the smaller the margin error the better the quality of the survey, even though this is not true. Many factors affect the quality of the survey, such as the method used, sampling, etc.
If you like the article we can follow it through the Random Creative Blog Feed or subscribe to get information from us.
